Introducing the Hardy Weinberg Problems Answer Key, your definitive guide to unraveling the complexities of population genetics. Delve into the intricacies of the Hardy-Weinberg principle, exploring its applications and limitations in understanding genetic variation and evolution.
Within these pages, you’ll embark on a journey through the Hardy-Weinberg equilibrium, gaining insights into the conditions that maintain genetic stability and the factors that can disrupt it. Discover how this principle serves as a cornerstone in population genetics, shedding light on the dynamics of genetic diversity and the forces that shape it.
Hardy-Weinberg Principle

The Hardy-Weinberg principle, also known as the Hardy-Weinberg equilibrium, is a fundamental concept in population genetics that describes the theoretical conditions under which allele and genotype frequencies in a population remain constant from one generation to the next. It is named after Godfrey Harold Hardy and Wilhelm Weinberg, who independently developed the principle in 1908.
The Hardy-Weinberg principle is based on the assumption that a population is large, random mating occurs, and there is no selection, mutation, gene flow, or genetic drift. Under these conditions, the allele and genotype frequencies will remain constant from generation to generation, and the population is said to be in Hardy-Weinberg equilibrium.
Conditions for Hardy-Weinberg Equilibrium, Hardy weinberg problems answer key
- The population must be large.
- Random mating must occur.
- There must be no selection.
- There must be no mutation.
- There must be no gene flow.
- There must be no genetic drift.
Hardy-Weinberg Problems
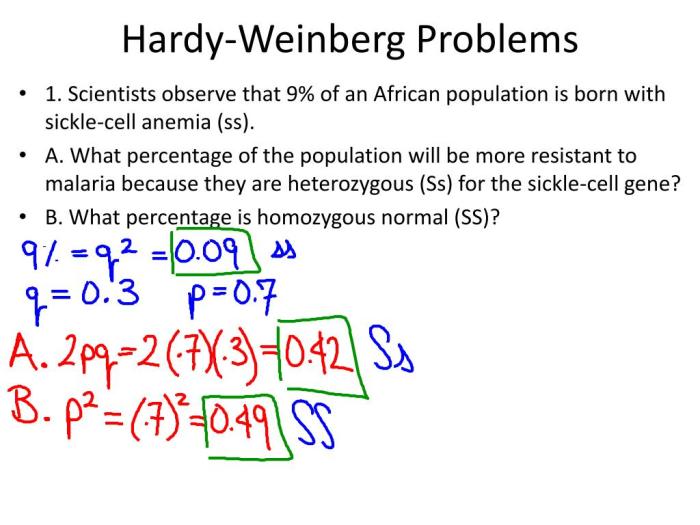
Hardy-Weinberg problems are used to calculate the allele and genotype frequencies in a population that is in Hardy-Weinberg equilibrium. The following steps can be used to solve Hardy-Weinberg problems:
- Determine the allele frequencies.
- Use the allele frequencies to calculate the genotype frequencies.
- Check to see if the population is in Hardy-Weinberg equilibrium.
The following table shows the allele and genotype frequencies at equilibrium for a single-locus, two-allele system:
| Allele | Frequency |
|---|---|
| p | 0.5 |
| q | 0.5 |
| Genotype | Frequency |
|---|---|
| pp | 0.25 |
| pq | 0.5 |
| 0.25 |
Applications of Hardy-Weinberg Principle
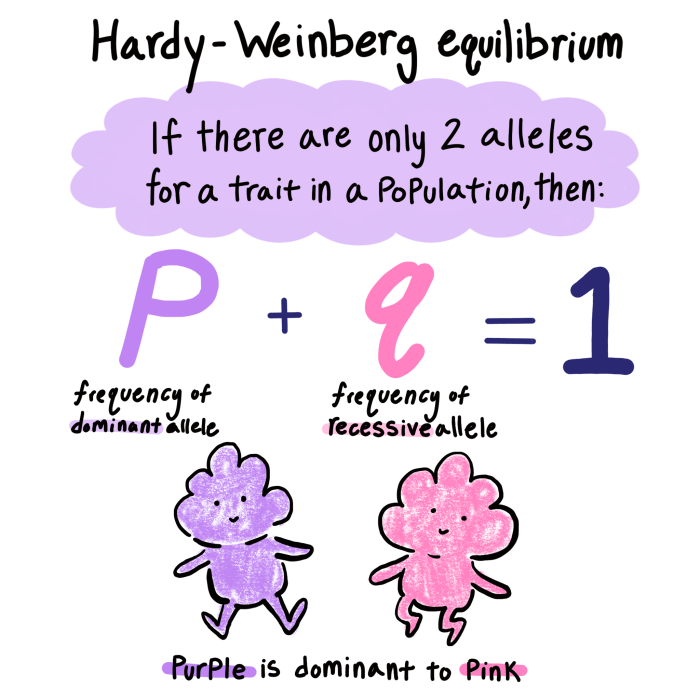
The Hardy-Weinberg principle has a number of applications in population genetics. It can be used to:
- Study genetic variation
- Predict the frequency of genetic diseases
- Monitor the effects of selection
- Estimate the effective population size
Limitations of Hardy-Weinberg Principle
The Hardy-Weinberg principle is a useful tool for understanding population genetics, but it is important to remember that it is only a theoretical model. In reality, most populations do not meet all of the assumptions of the Hardy-Weinberg principle. Non-random mating, mutation, gene flow, and genetic drift can all cause allele and genotype frequencies to deviate from Hardy-Weinberg equilibrium.
Commonly Asked Questions: Hardy Weinberg Problems Answer Key
What is the Hardy-Weinberg principle?
The Hardy-Weinberg principle describes the theoretical conditions under which the genetic variation in a population remains constant from generation to generation.
What are the conditions for Hardy-Weinberg equilibrium?
The conditions for Hardy-Weinberg equilibrium include: no mutation, no gene flow, random mating, and no natural selection.
How can the Hardy-Weinberg principle be used to study genetic variation?
The Hardy-Weinberg principle can be used to study genetic variation by comparing the observed allele and genotype frequencies in a population to the expected frequencies under Hardy-Weinberg equilibrium.
What are the limitations of the Hardy-Weinberg principle?
The limitations of the Hardy-Weinberg principle include the assumptions of no mutation, no gene flow, random mating, and no natural selection.